
I had no great revelation, after which I automatically would question anything in which I detected at least a whiff of the theoretical. I became a skeptic gradually. As time went by, more and more things that other people took for granted lost the ring of truth.
I especially became increasingly wary of categories. Not just the easily debunked categories which are formed by the manipulation of a bunch of selected qualities – things like group identifiers (Jewish, WASP, intellectual, extrovert), categories of taste (kitsch, sophisticated, low-brow), or aesthetic cubbyholing (modern, romantic, impressionistic) – but the big categories, like good and evil, innocence and guilt, consciousness and the unconscious.
“The leaves are beautiful,” says one of the girls. We are sitting on the back porch of the Havener family’s summer cottage on the shores of Wappinger Creek.
I look at the scrubby thicket on the other side of the stream and see thousands, hundreds of thousands, of bits of vegetation, each with its unique shape and its unique shade of green or, occasionally, of yellow, red or brown. Those also lay here and there on the small bit of lawn between the porch and the creek.
“What are “leaves?” I ask.
The question does not take anyone by surprise. I simply had started a round of another mind game.
“A leaf is the photosynthesizing component of vegetation,” says William.
I point to the blades of grass in the lawn. “So those are also leaves?”
“A leaf is a photosynthesizing component which grows from a stem, not directly from the ground,” says Micky Rudnick.
I think “Leaves of Grass,” but decide not to show off.
Lauren Havener can’t resist. “Leaves of Grass,” she says.
The rest of us groan.
I point to a tall dandelion near the porch. “Those green things then, that are growing are directly from the ground. They’re not dandelion leaves? ”
“‘Leaves’ is a general term,” says Mikey Stern.
“Exactly,” I say.
“Everything is in the mind, it’s all a matter of perception,” says William. That is the equivalent of saying “uncle.” I have triumphed without even having to bring in the dead, post-photosynthezition, leaves on the lawn, which I have been holding in reserve.
“But what’s beautiful?” says Tommy Oman.
Again, everyone groans. We’re bored with that game.
My tendency to concentrate on the uniqueness of things and disregard their collectivity lead to strenuous and sometimes fraught discussions with Tommy Oman, who felt he had to defend the honor of science against my depredations. Tommy was smarter than I and knew much more about math and science than I, so I relished his frustration when, despite his intelligence and knowledgeability, he had no answer when I held that there was no way to scientifically predict an event based on previous events, because no two events are identical. All he could do was sputter at my deliberate and confident stupidity.
This conflict once flared on the floor of a penthouse overlooking the Hudson, on Riverside Drive in New York City. I was a Freshman at Columbia, Tom (Tommy no more), a year behind me, had come down from Poughkeepsie to spend the weekend with his cousin, Michael, and Michael’s wife, Ruth.
Michael and Ruth were not only ultra-intelligent, ultra-cultured, ultra-sophisticated, but so remarkably affluent (remarkable for a young couple related to the gemütlich, proletarian Omans of Poughkeepsie) that they owned a country house in Connecticut as well as being able to afford the rent for a Riverside Drive penthouse.
Michael had taken it upon himself to refine the musical taste of us rubes from Poughkeepsie, for whom the pinnacle of Beethoven’s art was his orchestral works, by immersing us in the piano sonatas, interpreted by Schnabel, Backhaus, Gulda, Badura-Skoda, and played through Bozak speakers that must have cost more than $100 each. (Michael may have decided, correctly, that we weren’t ready for the quartets.)
Tom and I sat on the carpet, imbibing high culture and Pimm’s Cup (it is difficult now to reconcile Michael and Ruth’s urbane sophistication with their predilection for Pimm’s Cup). High culture, that day, consisted of Michael’s ruminations on an Edvard Munch show he had just seen at the Modern. (We had not yet entered the age of acronyms; the dreadful “MOMA” was still in the future.)
We began to discuss the degree to which art is autobiographical. Michael mentioned Dostoevsky's The Gambler. That Dostoevsky had a gambling problem was news to me - and I had thought myself a Dostoevsky aficionado. I nodded knowingly and speculated that the etiology of Dostoevsky's obsession may have been a desire to relive the euphoria he must have felt at his last minute reprieve from the firing squad. (That much I did know.)
Michael reminded us that plenty of people who had not faced a firing squad were obsessed with gambling. He characterized the excitement of gambling as a leap into the unknown.
"It’s not, if you know the probabilities," said Tom. "If you know the probabilities, gambling is just a math problem.”
I especially became increasingly wary of categories. Not just the easily debunked categories which are formed by the manipulation of a bunch of selected qualities – things like group identifiers (Jewish, WASP, intellectual, extrovert), categories of taste (kitsch, sophisticated, low-brow), or aesthetic cubbyholing (modern, romantic, impressionistic) – but the big categories, like good and evil, innocence and guilt, consciousness and the unconscious.
“The leaves are beautiful,” says one of the girls. We are sitting on the back porch of the Havener family’s summer cottage on the shores of Wappinger Creek.
I look at the scrubby thicket on the other side of the stream and see thousands, hundreds of thousands, of bits of vegetation, each with its unique shape and its unique shade of green or, occasionally, of yellow, red or brown. Those also lay here and there on the small bit of lawn between the porch and the creek.
“What are “leaves?” I ask.
The question does not take anyone by surprise. I simply had started a round of another mind game.
“A leaf is the photosynthesizing component of vegetation,” says William.
I point to the blades of grass in the lawn. “So those are also leaves?”
“A leaf is a photosynthesizing component which grows from a stem, not directly from the ground,” says Micky Rudnick.
I think “Leaves of Grass,” but decide not to show off.
Lauren Havener can’t resist. “Leaves of Grass,” she says.
The rest of us groan.
I point to a tall dandelion near the porch. “Those green things then, that are growing are directly from the ground. They’re not dandelion leaves? ”
“‘Leaves’ is a general term,” says Mikey Stern.
“Exactly,” I say.
“Everything is in the mind, it’s all a matter of perception,” says William. That is the equivalent of saying “uncle.” I have triumphed without even having to bring in the dead, post-photosynthezition, leaves on the lawn, which I have been holding in reserve.
“But what’s beautiful?” says Tommy Oman.
Again, everyone groans. We’re bored with that game.
My tendency to concentrate on the uniqueness of things and disregard their collectivity lead to strenuous and sometimes fraught discussions with Tommy Oman, who felt he had to defend the honor of science against my depredations. Tommy was smarter than I and knew much more about math and science than I, so I relished his frustration when, despite his intelligence and knowledgeability, he had no answer when I held that there was no way to scientifically predict an event based on previous events, because no two events are identical. All he could do was sputter at my deliberate and confident stupidity.
This conflict once flared on the floor of a penthouse overlooking the Hudson, on Riverside Drive in New York City. I was a Freshman at Columbia, Tom (Tommy no more), a year behind me, had come down from Poughkeepsie to spend the weekend with his cousin, Michael, and Michael’s wife, Ruth.
Michael and Ruth were not only ultra-intelligent, ultra-cultured, ultra-sophisticated, but so remarkably affluent (remarkable for a young couple related to the gemütlich, proletarian Omans of Poughkeepsie) that they owned a country house in Connecticut as well as being able to afford the rent for a Riverside Drive penthouse.
Michael had taken it upon himself to refine the musical taste of us rubes from Poughkeepsie, for whom the pinnacle of Beethoven’s art was his orchestral works, by immersing us in the piano sonatas, interpreted by Schnabel, Backhaus, Gulda, Badura-Skoda, and played through Bozak speakers that must have cost more than $100 each. (Michael may have decided, correctly, that we weren’t ready for the quartets.)
Tom and I sat on the carpet, imbibing high culture and Pimm’s Cup (it is difficult now to reconcile Michael and Ruth’s urbane sophistication with their predilection for Pimm’s Cup). High culture, that day, consisted of Michael’s ruminations on an Edvard Munch show he had just seen at the Modern. (We had not yet entered the age of acronyms; the dreadful “MOMA” was still in the future.)
We began to discuss the degree to which art is autobiographical. Michael mentioned Dostoevsky's The Gambler. That Dostoevsky had a gambling problem was news to me - and I had thought myself a Dostoevsky aficionado. I nodded knowingly and speculated that the etiology of Dostoevsky's obsession may have been a desire to relive the euphoria he must have felt at his last minute reprieve from the firing squad. (That much I did know.)
Michael reminded us that plenty of people who had not faced a firing squad were obsessed with gambling. He characterized the excitement of gambling as a leap into the unknown.
"It’s not, if you know the probabilities," said Tom. "If you know the probabilities, gambling is just a math problem.”
I pointed out that in a game of cards you can figure out probabilities from what cards have been played and what cards are left, but in Dostoevsky's game, roulette, while you can figure out your odds of winning, you can never predict where the ball is going to land, no matter how often the wheel is spun.
"Well, at least you know that if it was 23 the last time, there is less chance of it being 23 again, instead of some other number." said Tom.
"Well, at least you know that if it was 23 the last time, there is less chance of it being 23 again, instead of some other number." said Tom.
"No," I said, "There is more chance of it being 23 than some other number."
Tom scoffed. We had had this conversation many times before. I was happy, though, to see Michael, for all his sophistication, register a tic of surprise.
"Whatever circumstances existed to make the ball drop into the 23 slot will still exist when the wheel is spun the next time," I explained.
"Probably," said Tom.
"Fair enough," I said. "Probably."
I had won a point, but this was not a game. Tom and I were deeply invested in this question. To be melodramatic about it, how we were to lead the rest of our lives depended on it.
Michael still looked puzzled.
I continued. "The tabletop may not be absolutely horizontal; it probably slopes a teeny bit in one direction or another. The ridges separating the grooves can't all be exactly the same height; some must be a little higher, some a little lower. The ball can't be a perfect sphere. These are small details, but they must have influenced the outcome, which was the ball stopping on 23. They'll still exist when the wheel is spun again.”
"But the real world is all we have. All natural laws, all science is based on the real world - even the laws of gravity." Tom could not keep from sounding a little plaintive.
"Not the science of mathematics, not logic," I pressed. “They are not based on the real world; they are based on the ideal, Platonic world. It's illogical to say that in the ideal world – if the wheel lay on the absolute horizontal, if its parts were cut with absolute precision, if the ball were a perfect sphere, if it were all made of a material harder than diamonds – there is less chance that a number will repeat itself than that some other particular number will win. The chances are equal. With every roll, the chances always equal."
"No,” said Tom. “According to the law of probabilities, every other number has a better chance of winning than 23 has."
"Then the law of probabilities is an ass," I said.
We were at our usual impasse. Each of us thought the other was being purposively dense. In truth, we had arrived at an antimony where – like arguments over the existence of God, mind-body dualism, whether or not the Good was relative, and free will vs. predestination – any further discussion became a game. It usually devolved into a game of semantics, but this time Tom said, "Let's roll a die and see who’s right.”
Michael produced a felt-lined leather cup and five large Bakelite dice which, he volunteered, he'd bought from the bartender of a taberna in a village in Andalucía.
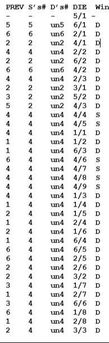
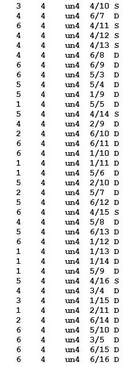
We removed four of the dice and, pencil and paper at hand, began to toss the remaining one.
According to the laws of probability, that is, according to Tom, there was less chance that a number would be repeated than that one of the other five numbers would appear. According to my theory that every event is a discrete occurrence, there was the same chance – one in six – of a number repeating itself as there was of another number appearing. That was in a perfect world. According to my theory that every event is unique, in the real world, in which there was a hand-made Spanish leather dice cup and a presumably often-tumbled Bakelite die, one face of the die was more likely to turn up than any of the other five faces.
Our game did not keep Michael from that afternoon’s project of comparing Backhaus’ performance of Opus 10, No. 3, with Gulda’s. The rhythmic muffled beat of the die rolling out of the felt-lined cup onto the carpet accompanied their playing like the drumroll from a distant marching army.
I cannot remember which of us was ahead when it was time for us all walk over to the Empire Szechuan on Broadway for dinner.
Michael and Ruth, who were habitués, ordered off-menu, of course.
Tom scoffed. We had had this conversation many times before. I was happy, though, to see Michael, for all his sophistication, register a tic of surprise.
"Whatever circumstances existed to make the ball drop into the 23 slot will still exist when the wheel is spun the next time," I explained.
"Probably," said Tom.
"Fair enough," I said. "Probably."
I had won a point, but this was not a game. Tom and I were deeply invested in this question. To be melodramatic about it, how we were to lead the rest of our lives depended on it.
Michael still looked puzzled.
I continued. "The tabletop may not be absolutely horizontal; it probably slopes a teeny bit in one direction or another. The ridges separating the grooves can't all be exactly the same height; some must be a little higher, some a little lower. The ball can't be a perfect sphere. These are small details, but they must have influenced the outcome, which was the ball stopping on 23. They'll still exist when the wheel is spun again.”
"But the real world is all we have. All natural laws, all science is based on the real world - even the laws of gravity." Tom could not keep from sounding a little plaintive.
"Not the science of mathematics, not logic," I pressed. “They are not based on the real world; they are based on the ideal, Platonic world. It's illogical to say that in the ideal world – if the wheel lay on the absolute horizontal, if its parts were cut with absolute precision, if the ball were a perfect sphere, if it were all made of a material harder than diamonds – there is less chance that a number will repeat itself than that some other particular number will win. The chances are equal. With every roll, the chances always equal."
"No,” said Tom. “According to the law of probabilities, every other number has a better chance of winning than 23 has."
"Then the law of probabilities is an ass," I said.
We were at our usual impasse. Each of us thought the other was being purposively dense. In truth, we had arrived at an antimony where – like arguments over the existence of God, mind-body dualism, whether or not the Good was relative, and free will vs. predestination – any further discussion became a game. It usually devolved into a game of semantics, but this time Tom said, "Let's roll a die and see who’s right.”
Michael produced a felt-lined leather cup and five large Bakelite dice which, he volunteered, he'd bought from the bartender of a taberna in a village in Andalucía.
We removed four of the dice and, pencil and paper at hand, began to toss the remaining one.
According to the laws of probability, that is, according to Tom, there was less chance that a number would be repeated than that one of the other five numbers would appear. According to my theory that every event is a discrete occurrence, there was the same chance – one in six – of a number repeating itself as there was of another number appearing. That was in a perfect world. According to my theory that every event is unique, in the real world, in which there was a hand-made Spanish leather dice cup and a presumably often-tumbled Bakelite die, one face of the die was more likely to turn up than any of the other five faces.
Our game did not keep Michael from that afternoon’s project of comparing Backhaus’ performance of Opus 10, No. 3, with Gulda’s. The rhythmic muffled beat of the die rolling out of the felt-lined cup onto the carpet accompanied their playing like the drumroll from a distant marching army.
I cannot remember which of us was ahead when it was time for us all walk over to the Empire Szechuan on Broadway for dinner.
Michael and Ruth, who were habitués, ordered off-menu, of course.
 RSS Feed
RSS Feed